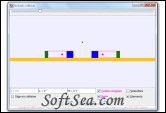
Analyze an inelastic collision with this application. Inelastic Collision of Particles with Structure model displays the inelastic collision between two equal "particles" with structure on a smooth horizontal surface. Each particle has two microscopic elements which interact through a massless spring of stiffness k and natural length L. The mass of one of the microscopic elements and the spring length of the connector spring can be changed via textboxes. You can modify this simulation if you have Ejs installed by right-clicking within the plot and selecting "Open Ejs Model" from the pop-up menu item.

Each "particle" has two "microscopic" elements of masses m and am, interacting through a massless spring of stiffness k and natural length L and they move on a smooth horizontal surface.
Initially the spring is unstretched and the two "particles" move with opposite velocities of magnitude v.At some point the two "particles" collide.
The collision between the two inner masses is elastic; but, since the oscillatory mode is excited, one can understand (from a "macroscopic" point of view) the transfer from the translational mode (identified with the motion of the center-of-mass) to the oscillatory mode as a transfer of kinetic energy to an internal elastic mode.
1. The unit time is (m/k)1/2 .
2. The unit length is v (m/k)1/2 .

You can select the values for the parameters a and L, as well as the interval between animation frames (?t). Press Enter after modifying these values.
You can display the whole "particle" and/or its "microscopic" elements by checking Body and/or Elements.
You can also display, optionally, the center-of-mass, as well as its velocity and those of the "microscopic" elements.
Use the slider on the top of the window to select the displayed range.
After each collision the program will display the coefficient of restitution (e = V / |v|, where V is the velocity of the center-of-mass). With this definition e will be negative if the center-of-mass is approaching the center.

Requirements:
* Java

The license of this software is Free, you can free download and free use this graphing software.